Question

A flow of 12 m/s passes through a 6 m wide, 2 m deep rectangular channel with a bed slope of 0. 001. If the mean velocity of flow is 1 m/s, what is the Manning's coefficient?

Answer 1

manning's coefficient is 0.0357

Step-by-step explanation:

Given:

Velocity of flow, v = 12 m/s

Width of the channel, b = 6 m

Depth of the channel, d = 2 m

bed slope, s = 0.001

mean velocity of flow, V = 1 m/s

now, the velocity is given as:

`V= (1)/(n)R^{(2)/(3)}S^{(1)/(2)}`

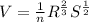
where,

n is the manning's coefficient

R is hydraulic mean depth

R = (Area of the channel) / (wetted Perimeter of the channel)

now,

R = (2 × 6) / ((2 × 2) + 6)

or

R = 12 / 10 = 1.2 m

now, on substituting the values in the equation for velocity, we get

`1= (1)/(n)1.2^{(2)/(3)}(0.001)^{(1)/(2)}`

or

`n= 1.2^{(2)/(3)}(0.001)^{(1)/(2)}`

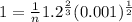
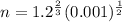
or

n = 0.0357

hence, the value of manning's coefficient is 0.0357