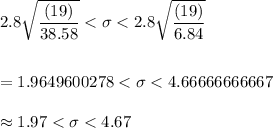Answer:
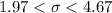
Explanation:
The confidence interval for population standard deviation is given by :-
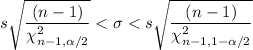
Given : Sample size :

Standard deviation :

Significance level :
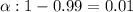
Critical value using chi-square distribution table :
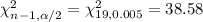
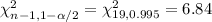
Then , 99% confidence interval for the standard deviation of the replacement times of all washing machines of this type will be :