Question

Solve x2 + 10x = 24 by completing the square. Which is the solution set of the equation?

1-5 - 734,-5 + 734)
02-5 - 129, -5 +129) |
0 (-12, 2}
0 (-2,12}
Search Windows

Answer 1

(- 12, 2 )

Explanation:

Given

x² + 10x = 24

To complete the square

add ( half the coefficient of the x- term )² to both sides

x² + 2(5)x + 25 = 24 + 25

(x + 5)² = 49 ( take the square root of both sides )

x + 5 = ±
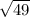`√(49)` = ± 7 ( subtract 5 from both sides )

x = - 5 ± 7

Hence

x = - 5 - 7 = - 12 or x = - 5 + 7 = 2

Solution is ( - 12, 2 )

Answer 2

(-12, 2).

Explanation:

x^2 + 10x = 24

Divide the + 10 by 2 to give the following identity:

x^2 + 10x is equivalent to (x + 5)^2 - 25 so:

(x + 5)^2 - 25 = 24

(x + 5)^2 = 49

Taking square roots:

x + 5 = +/- 7.

x = -5 + 7 = 2

and x = -5 - 7 = -12.