Question

What is the soultion to the system of equations 2x+y-z=-3, 5x-2y+2z=24,3x-z=5

(4, 1, 8)

(−1, 0, −8)

(−5, 4, −3)

(2, −6, 1)

Answer 1

(2,-6,1)

Explanation:

y = -2x + z - 3

5x - 2•(-2x+z -3) + 2z = 24

9x = 18

9x = 18

x = 2

3•(2) - z = 5

- z = -1

z = 1

x = 2

y = -2x+z-3

z = 1

y = -2(2)+(1)-3 = -6

{x,y,z} = {2,-6,1}

Answer 2

Answer: The correct option is

(D) (2, -6, 1).

Step-by-step explanation: We are given to solve the following system of linear equations :

`2x+y-z=-3~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)\\\\5x-2y+2z=24~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(ii)\\\\3x-z=5~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(iii)`

From equation (iii), we have

`3x-z=5\\\\\Rightarrow z=3x-5~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(iv)`

Substituting the value of z from equation (iv) in equations (i) and (ii), we have

`2x+y-(3x-5)=-3\\\\\Rightarrow -x+y+5=-3\\\\\Rightarrow x-y=8~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(v)`

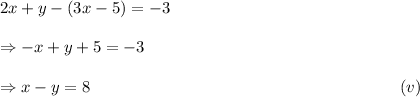
and

`5x-2y+2(3x-5)=24\\\\\Rightarrow 11x-2y=34~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~(vi)`

(v) × 2 - (vi) gives us

`2(x-y)-(11x-2y)=16-34\\\\\Rightarrow -9x=-18\\\\\Rightarrow x=2.`

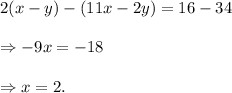
So, from equation (v), we get

`2-y=8\\\\\Rightarrow y=2-8\\\\\Rightarrow y=-6.`

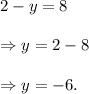
From (iv), we get

`3* 2-5=z\\\\\Rightarrow z=1.`

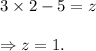
Thus, the required solution is

(x, y, z) = (2, -6, 1).

Option (D) is CORRECT.