Question

The amounts a soft drink machine is designed to dispense for each drink are normally distributed, with a mean of 12.1 fluid ounces and a standard deviation of 0.3 fluid ounce. A drink is randomly selected.

a) Find the probability that the drink is less than 11.9 fluid ounces (round to four decimal places as needed).
b) Find the probability that the drink is between 11.6 and 11.9 fluid ounces (round to four decimal places as needed).
c) Find the probability that the drink is more than 12.6 fluid ounces (round to four decimal places as needed)
d) Is the drink containing more than 12.6 fluid ounces an unusual event?
A.No, because the probability that a drink containing more than 12.6 fluid ounces is greater than 0.05, this event is not unusual.
B.No, because the probability that a drink containing more than 12.6 fluid ounces is less than 0.05, this event is not unusual.
C.yes, because the probability that a drink containing more than 12.6 fluid ounces is greater than 0.05, this event is unusual.
D.Yes, because the probability that a drink containing more than 12.6 fluid ounces is less than 0.05, this event is unusual.

Answer 1

`\mu = 12.1\\\sigma = 0.3`

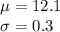
a) Find the probability that the drink is less than 11.9 fluid ounces

We are supposed to find P(x<11.9)

x= 11.9

We will use z score formula :

`z=(x-\mu)/(\sigma)`

`z=(11.9-12.1)/(0.3)`

`z=-0.67`

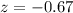
Refer the z table

So, P(z<-0.67) = 0.2514

Hence the probability that the drink is less than 11.9 fluid ounces is 0.2514

b)Find the probability that the drink is between 11.6 and 11.9 fluid ounces

x= 11.6

We will use z score formula :

`z=(x-\mu)/(\sigma)`

`z=(11.6-12.1)/(0.3)`

`z=-1.67`

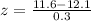
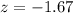
x= 11.9

We will use z score formula :

`z=(x-\mu)/(\sigma)`

`z=(11.9-12.1)/(0.3)`

`z=-0.67`

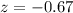
We are supposed to find P(11.6<x<11.9)

So, P(11.6<x<11.9)= P(x<11.9)-P(x<11.6)

P(-1.67<z<-0.67)= P(z<-0.67)-P(z<-1.67)

Refer the z table

P(-1.67<z<-0.67)= P(z<-0.67)-P(z<-1.67)

P(-1.67<z<-0.67)= 0.2514-0.0475

P(-1.67<z<-0.67)= 0.2039

So, the probability that the drink is between 11.6 and 11.9 fluid ounces is 0.2039.

c)Find the probability that the drink is more than 12.6 fluid ounces

We are supposed to find P(x>12.6)

x= 12.6

We will use z score formula :

`z=(x-\mu)/(\sigma)`

`z=(12.6-12.1)/(0.3)`

`z=1.67`

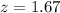
Refer the z table

P(x>12.6)=1-P(x<12.6)

P(z>1.67)=1-P(z<1.67)

P(z>1.67)=1-0.9525

P(z>1.67)=0.0475

Hence the probability that the drink is more than 12.6 fluid ounces is 0.0475

d) Is the drink containing more than 12.6 fluid ounces an unusual event?

Since p value is less than alpha (0.05)

Answer = No, because the probability that a drink containing more than 12.6 fluid ounces is less than 0.05, this event is not unusual.