Question

A 4.00-kg particle moves along the x axis. Its position varies with time according to x 5 t 1 2.0t 3, where x is in meters and t is in seconds. Find (a) the kinetic energy of the particle at any time t, (b) the acceleration of the particle and the force acting on it at time t, (c) the power being delivered to the particle at time t, and (d) the work done on the particle in the interval t 5 0 to t 5 2.00 s

Answer 1

Step-by-step explanation:

Given equation is ,

x =t + 2 t³ ,

dx/dt = velocity ( v ) = 1 + 6 t²

a) kinetic energy = 1/2 m v² = .5 x 4 ( 1 + 6 t² )² = 2 ( 1 + 6 t²)²

b ) Acceleration = dv /dt = 12 t .

force( F ) = mass x acceleration = 4 x 12 t = 48 t

Power = force x velocity = 48 t x ( 1 + 6 t²). = 48 t + 288 t³ )

work done = ∫ F dx =∫ 48 t x( 1 + 6t² )dt ; = [48t²/2 + 48 x 6 x t³ /3 = 24 t² + 96 t³ )]₀² = 864 J

Answer 2

Givens:

• 
  `x=t+2.0t^(3)`
• 
  `m=4.00 \ kg`

We know that kinetic energy is:

`K=(1)/(2)mv^(2)`

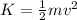
So, we just need to calculate the speed. We have the equation of the movement, if we derivate that expression, we'll have the speed:

`(dx)/(dt)=v\\ v=(d)/(dt)(t+2.0t^(3))\\v=1+2(3)t^(2) \\v=6t^(2)+1`

Which is the speed at any time t.

Now, we replace the expression to find the kinetic energy at any time t:

`K=(1)/(2)m(6t^(2)+1)^(2)`

`K=(1)/(2)(4)(36t^(4)+12t^(2)+1)\\K=2(36t^(4)+12t^(2)+1)\\K=72t^(4)+24t^(2)+2`

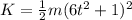
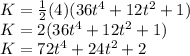
So, this is the kinetic energy at energy at any time t.

Through derivation we can find the acceleration and force at any time t:

`a=(dv)/(dt)\\a=(d)/(dt)(6t^(2)+1)\\a=12t`

Also, we know that
`F=ma`

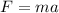
Replacing values:
`F=4(12t)=48t`

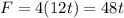
The power is define as the product of the force and the velocity:

`P=Fv=48t(6t^(2)+1)=288t^(3)+48`

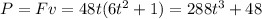
At last, we now that the work is define as:
`W=\int Fdx`

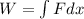
So, we just replace the force, and integrate it between t=0 and t=2 sec.

`W=\int _(0)^(2) 48t(6t^(2)+1)dt\\W=\int_(0)^(2) (288t^(3)+48t)dt\\W= (288t^(4) )/(4)+(48t^(2))/(2) ]_(0)^(2) \\W= 72t^(4)+24t^(2)]_(0)^(2)\\W=72(2)^(4)+24(2)^(2)\\W= 1152 + 96=1248 \ J`