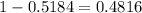Answer:
A consumer organization estimates that over a 1-year period 16% of cars will need to be repaired once, 9% will need repairs twice, and 3% will require three or more repairs..
We have the cars that do not need repair =
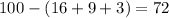 %
%
(16+9+3=28% cars need repair of some sort)
What is the probability that :
a) neither will need repair?
=>
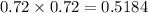
b) both will need repair?
=>

c) at least one car will need repair?
=>

=>