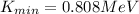Answer:
The minimum kinetic energy of the particle,
Step-by-step explanation:
In this question we have given,
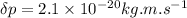
mass, m=
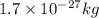
we have to find the minimum kinetic energy of the particle,
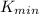 =?
=?
We know that momentum and velocity are related by following formula,
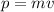
Therefore
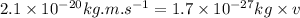
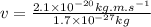
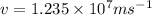
Now we know that kinetic energy is given as

Therefore minimum kinetic energy is given as
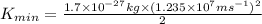
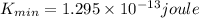
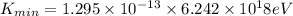

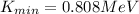
The minimum kinetic energy of the particle