Answer:
72 leaps
Explanation:
Given: A dog chasing a rabbit, rabbit runs 60 leaps before the dog. When the dog jumps 2 leaps rabbit can jumps 3 leaps. But rabbit have to jump 7 leaps to cover the distance that dog cover by 3 leaps.
To Find: number of leaps that the dog jumps when rabbit and dog meet.
Solution:
Let the units of leaps jumped by rabbit be r
Let the units of leaps jumped by dog be d
7 leaps of rabbit =3 leaps of dog
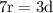

rabbit runs 60 leaps before the dog,
therefore
distance between dog and rabbit=60r=

When the dog jumps 2 leaps rabbit can jump 3 leaps
speed of rabbit(
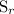 )=
)=
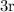 =
=
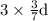
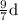
speed of dog(
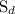 ) =
) =

As dog and rabbit are running is same direction,
relative speed of dog and rabbit (
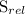 )=speed of dog-speed of rabbit
)=speed of dog-speed of rabbit
(
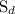 )-(
)-(
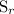 )
)

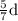
the distance to be covered by dog before catching rabbit

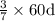
Now,
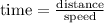
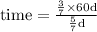

leaps jumped by dog in
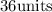 of time
of time
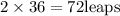
Hence the leaps jumped by dog when dog and rabbit meet are 72