Question

A rifle is a long-barreled firearm which imparts a high velocity to a small ballistic, called a bullet, by exploding a charge behind the bullet. A rifle is typically 6 kg, and the bullet is 12 grams. The velocity of the bullet upon leaving the barrel is 300 m/s. Take the system to mean the combination of rifle and bullet. What is the momentum of the system, bullet and rifle just after the bullet leaves the barrel?

Answer 1

The momentum of the system, bullet and rifle just after the bullet leaves the barrel is zero, 3.6 kg m/s and -3.6 kgm/s respectively

Step-by-step explanation:

Given that,

Mass of the rifle = 6 kg

Mass of the bullet = 12 g

Velocity of bullet = 300 m/s

We need to calculate the momentum of bullet

Using formula of momentum

`P = mv`

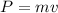
Where, m = mass of bullet

v = velocity of bullet

Put the value into the formula

`P=12*10^(-3)*300`

`P=3.6\ kg m/s`

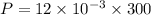
The momentum of the bullet is 3.6 kgm/s.

The momentum of rifle is opposite direction of momentum of bullet and equal in magnitude.

The momentum of rifle = - 3.6 kgm/s

Therefore, The momentum of system must be zero.

Hence, The momentum of the system, bullet and rifle just after the bullet leaves the barrel is zero, 3.6 kg m/s and -3.6 kgm/s respectively.