Answer:
8.1 x 10³ m
Step-by-step explanation:
D = diameter of the aperture =3 mm = 0.003 m
x = distance between the two headlights = 1.80 m
λ = wavelength of the visible spectrum = 550 x 10⁻⁹ m
d = distance of the headlights from eyes = ?
Using Reyleigh's criterion,
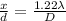
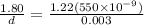
d = 8047.7 m
d = 8.1 x 10³ m