Answer:
- 1
Explanation:
z₁ - z₂ = 1 - i - (- 2 + 4i) = 1 - i + 2 - 4i = 3 - 5i, thus

Rationalise the denominator by multiplying the numerator/denominator by the complex conjugate of the denominator
The conjugate of 1 - i is 1 + i, so
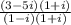
expand numerator / denominator noting i² = - 1
=
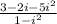
=
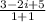
=

= 4 - i
Thus Im [
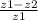 ] = - 1
] = - 1