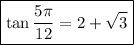Answer:
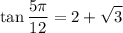
Explanation:
Tangent Half Angle
Given an angle θ, then:

We are required to find:
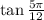
But it cannot be found in tables of main angles. We can use the angle

And use the formula above to find the required operation. Hence:
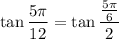

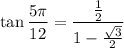
Operating:

Simplifying:
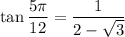
Rationalizing:
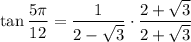
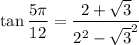
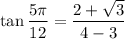
Finally: