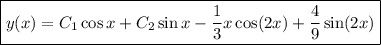The corresponding homogeneous ODE has characteristic equation
 with roots at
with roots at
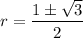 , thus admitting the characteristic solution
, thus admitting the characteristic solution
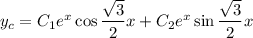
For the particular solution, assume one of the form
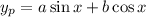
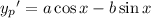

Substituting into the ODE gives

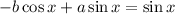
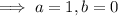
Then the general solution to this ODE is
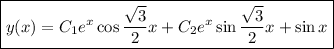
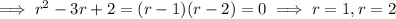
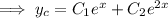
Assume a solution of the form

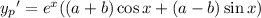
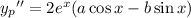
Substituting into the ODE gives

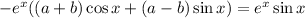
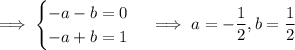
so the solution is
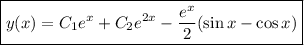
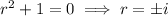
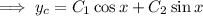
Assume a solution of the form
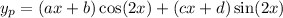
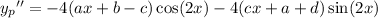
Substituting into the ODE gives
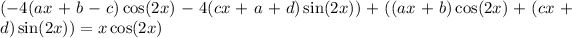
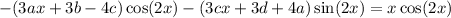
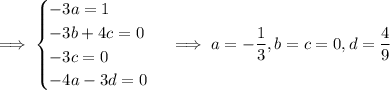
so the solution is