Question

AP Stats The College Board reported that 57.9% of all students who took the 2013 AP Statistics exam earned scores of 3 or higher. One teacher wondered if the perfor- mance of her school was better. She believed that year’s students to be typical of those who will take AP Stats at that school and was pleased when 34 of her 54 students achieved scores of 3 or better. Can she claim that her school is better?

Answer 1

There is no sufficient evidence to support her claim that her school is better

Explanation:

Consider the provided information.

She believed that year’s students to be typical of those who will take AP Stats at that school and was pleased when 34 of her 54 students achieved scores of 3 or better.

So x = 34, the value of n is 54, p = 57.9% = 0.579

Now, determine the hypothesis as shown:

`H_0: p\leq 0.579\\H_a: p>0.579`

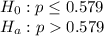
The sample proportion can be calculated as:

`\hat p = (x)/(n)\\\hat p=(34)/(54) \approx0.6296`

Now, determine the value of the test statistic:

`z=\frac{\hat p-p_0}{\sqrt{ (p_0(1-p_0))/(n)}}`

Substitute the respective value in above.

`z=\frac{0.6296-0.579}{\sqrt{ (0.579(1-0.579))/(54)}}`

`z=(0.0506)/(0.0672)`

`z=0.753`

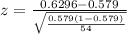
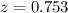
Now the P value is called the probability of obtaining the value from the test statistic. Use the P-value in table Z to find the P value of z=0.753

According to the P value table the value for z=0.753 is 0.226 or 22.6%.

If the P value of the provided question is less than the significance level, reject the null hypothesis:

P>0.05 thus, Fail to reject null hypotheses.

Thus, there is no sufficient evidence to support her claim that her school is better