Question

A nonconducting spherical shell of inner radius R 1 and outer radius R 2 contains a uniform volume charge density ρ throughout the shell. Use Gauss's law to derive an equation for the magnitude of the electric field at the following radial distances r from the center of the sphere. Your answers should be in terms of ρ, R 1, R 2, r, ε0, and π.

(a) r < R 1

Answer 1

E=0

Step-by-step explanation:

According to Gauss law:

`\int E.dA =(Q_(en))/(\epsilon_o)`

So, first calculate the amount of charge enclosed in the region where r < R₁.

Since, the volume charge density is just throughout the shell (i.e. between R₂ and R₁), there is no charge in the region r < R₁. Therefore,
`{Q_(en)=0`

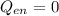
⇒ E = 0 for r < R₁