Question

The ratio of the number of Victor’s tools to the number of Ilya’s tools is 5:2. Victor has 42 more tools than Ilya. How many tools should Victor give to Ilya so that the ratio of the number of Victor’s tools to the number of Ilya’s tools will be 3:4

Answer 1

Victor gives 28 tools to IIya.

Explanation:

Consider the provided information

The ratio of the number of Victor’s tools to the number of Ilya’s tools is 5:2

Let V represents the Victor and I represent llya

Therefore,

`(V)/(I) = (5)/(2)`

It is given that Victor has 42 more tools than Ilya.

`(V)/(I) = (5)/(2)=(42+I)/(I)`

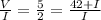
Solve the above equation.

`(5)/(2)=(42+I)/(I)`

`5I=84+2I`

`3I=84`

`I=28`

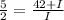
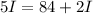
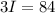
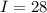
Thus, IIya has 28 tools.

Victor has 42 more tools than Ilya. Therefore 42 + 28 = 70.

Victor has 70 tools.

We want to find the number of tools victor needs to give to IIya so that the ratio of the number of Victor's tools to the number of Ilya’s tools will be 3:4

Let Victor gives x tools to IIya. Thus,

`(3)/(4)=(70-x)/(28+x)`

`3(28+x)=4(70-x)`

`84+3x=280-4x`

`7x=196`

`x=28`

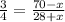
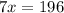
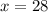
Hence, Victor gives 28 tools to IIya.