Answer:
The equivalent resistance of the combination is R/100
Step-by-step explanation:
Parallel Connection of Resistances
If resistances R1, R2, R3,...., Rn are connected in parallel, the equivalent resistance is calculated as follows:
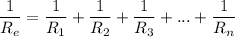
The electric resistance of a wire is directly proportional to its length. If a wire of resistance R is cut into 10 equal parts, then each part has a resistance of R/10.
It's known the 10 parts or resistance R/10 were connected in parallel, thus the electric resistance is:
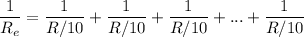
Note the sum consists of 10 equal terms. Operating on each term:
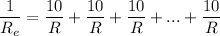
The sum of 10 identical fractions yields 10 times each fraction:
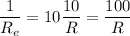
Solving for Re needs to take the reciprocal of both sides of the equation:
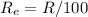
The equivalent resistance of the combination is R/100