Answer:
-5
Explanation:
Geometric sequences have common ratios.
You can see if a sequence is geometric by computing:
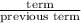 .
.
If you get the same result per consecutive pair, then there is a common ratio and the sequence is geometric.
Let's see:
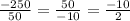
So if this equations holds (if all 3 fractions equal the same thing), then is geometric and we have also have computed the common ratio.
The first fraction results in -250/50=-5.
The second fraction results in 50/-10=-5.
The third fraction results in -10/2=-5.
All three fractions are equal and so the equation holds so it is geometric and the common ratio is -5.