Answer:
C.
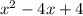
D.
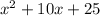
Explanation:
A trinomial
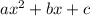 is perfect square trinomial if,
is perfect square trinomial if,
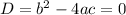 ,
,
A.

By comparing,
a = 1, b = 0, c = -9,
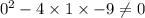
⇒ it is not a perfect square trinomial.
B.
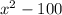
By comparing,
a = 1, b = 0, c = -100,
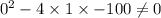
⇒ it is not a perfect square trinomial.
C.
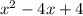
By comparing,
a = 1, b = -4, c = 4,

⇒ it is a perfect square trinomial.
D.
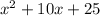
By comparing,
a = 1, b = 10, c = 25,
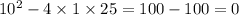
⇒ it is a perfect square trinomial.
E.
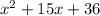
By comparing,
a = 1, b = 15, c = 36,
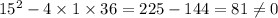
⇒ it is not a perfect square trinomial.