Question

For a certain frozen pizza, as the cost goes from $2 to $4, the demand can be modeled by the formula y = -10x2 + 60x + 180, where x represents the cost and y represents the number of pizzas sold. Estimate the cost that will result in the greatest demand.

Answer 1

The cost that will result in the greatest demand is $3

Explanation:

Let

x ------> represents the cost

y -----> represents the number of pizzas sold

we have

`y=-10x^(2)+60x+180`

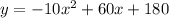
This is a quadratic equation (vertical parabola) open downward

The vertex is a maximum

To find out the greatest demand calculate the vertex

The equation of a vertical parabola in vertex form is equal to

`y=a(x-h)^(2)+k`

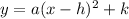
where

(h,k) is the vertex

Convert the quadratic equation in vertex form

Complete the squares

`y-180=-10x^(2)+60x`

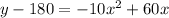
Factor the leading coefficient

`y-180=-10(x^(2)-6x)`

`y-180-90=-10(x^(2)-6x+9)`

`y-270=-10(x^(2)-6x+9)`

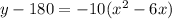
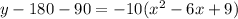
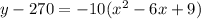
Rewrite as perfect square

`y-270=-10(x-3)^(2)`

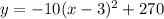`y=-10(x-3)^(2)+270` ----> equation in vertex form

The vertex is the point (3,270)

therefore

For the greatest demand

The cost is $3

The number of pizzas sold is 270