For this case we must simplify the following expression:
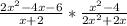
So, by rewriting we have:
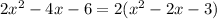
We factor the parenthesis trinomial by looking for two numbers that, when multiplied, are obtained -3 and when added together, -2 is obtained. These numbers are -3 and 1, so:
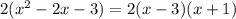
On the other hand we have to:
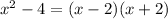
Last we have:
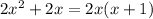
Thus, rewriting the expression:
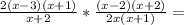
Simplifying:

Answer:
Option A