Answer:
option d) -9 J
Step-by-step explanation:
Given:
Mass, m = 3.0 kg
time, t = 6.0 seconds
Velocity of mass, v = 2.0 m/s
height, h = 2 m
Now, using the concept of work-Energy theorem
we have
Net work done = change in kinetic energy
or
Work done by gravity + work done by the friction = Final kinetic energy - Initial kinetic energy
mgh +
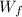 =
=
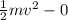
on substituting the values in the above equation, we get
3 × 9.8 × 2 +
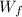 =
=
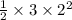
or
58.8 +
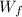 = 6
= 6
or
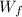 = -52.8 J
= -52.8 J
here negative sign depicts that the work is done against the motion of the mass
also,
Power = (Work done)/time
or
Power = -52.8/6 = -8.8 W ≈ 9 J
Hence, option d) -9 J is correct