Question

What is the domain and range of f(x) = (2/3)^x

Answer 1

Range: y>0

Domain is all real numbers.

Explanation:

The range of that function has to be positive, therefore it must be greater than 0. The domain is the x value and can be either positive or negative, thus the domain is all real numbers.

Answer 2

Domain = { all real values of x }

Range = { y > 0 }

Explanation:

Any exponential function of the form:

`y=a^x`

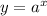
Where a is positive

• Will always go through the point (0,1)
• The domain would be defined for all real values and range would be greater than 0

But when a is negative

• The graph will go through (0,-1)
• The domain would be defined for all real values and range would be less than 0

So, we can see the function is the form where a is positive, hence

Domain = { all real values of x }

Range = { y > 0 }

Note: Graph of this function is shown in attached pic