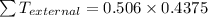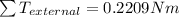Question

Two wheels have the same mass and radius of 4.4 kg and 0.48 m, respectively. One has (a) the shape of a hoop and the other (b) the shape of a solid disk. The wheels start from rest and have a constant angular acceleration with respect to a rotational axis that is perpendicular to the plane of the wheel at its center. Each turns through an angle of 14 rad in 8.0 s. Find the net external torque that acts on each wheel.

Answer 1

1)
`\sum T_(externalhoop)=0.4418Nm`

2)
`\sum T_(externaldisc)=0.2209Nm`

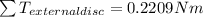
Step-by-step explanation:

Using the second equation of angular motion we have

`\theta =\omega _(o)t+(1)/(2)\alpha t^(2)`

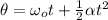
Since the wheels start from rest we ahve
`omega _(o)=0`

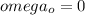
Applying the given values in the equation we have

`14=(1)/(2)\alpha * 8^(2)\\\\\therefore \alpha =(28)/(64)=0.4375rad/s^(2)`

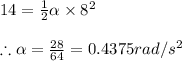
Now by newton's second law of motion in angular motion we have

`\sum T_(external)=I\alpha`

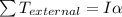
1) For Hoop We have

`I_(hoop)=Mr^(2)\\\\\therefore I_(hoop)=4.4*(0.48)^(2)=1.01376kgm^(2)`

Thus

`\sum T_(external)=1.01376* 0.4375`

`\sum T_(external)=0.4418Nm`

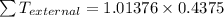
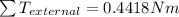
2)For disc We have

`I_(disc)=(Mr^(2))/(2)\\\\\therefore I_(hoop)=2.2*(0.48)^(2)=0.506kgm^(2)`

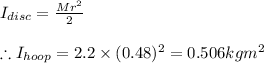
Thus

`\sum T_(external)=0.506* 0.4375`

`\sum T_(external)=0.2209Nm`