Answer:
Explanation:
Given ABCD is a parallelogram
To prove ⇒ AC trisects BD, and BD bisects AC.
Proof ⇒ Since coordinates of points A, B, C and D have been given in the diagram. If we prove that midpoint of AC and BD are common then AC and BD will equally bisect each other.
Midpoint of AC =
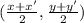
coordinates of A and C are (0,0) and (2a + 2b, 2c)
Now mid point
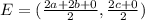
= [(a+b),c]
Now mid point of BD =
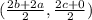
= [(b+a), c]
It proves that midpoints of AC and BD are common.
So AC trisects BD and BD bisects AC.