Answer:
The required probability is
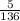 .
.
Step-by-step explanation:
Consider the provided information.
It is given that the total number of reference book is 2.
Fiction books are 10 and non fiction books are 5.
Thus, the total book are: 5 + 10 + 2 = 17
The probability that Nadia picks up a reference book is:

Now with out replacing it she pick up another book.
The probability that she randomly picks up a non-fiction book is:
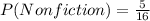
Now we need to find the probability that she randomly picks up a reference book and then, without replacing it, picks up a nonfiction book.
In order to find the required probability multiply both the probability as shown:
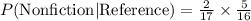
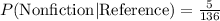
Hence, the required probability is
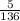 .
.