Answer:
0.0034 sec
Step-by-step explanation:
L = initial length
T = initial time period = 2.51 s
Time period is given as
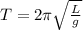
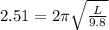
L = 1.56392 m
L' = new length
ΔT = Rise in temperature = 142 °C
α = coefficient of linear expansion = 19 x 10⁻⁶ °C
New length due to rise of temperature is given as
L' = L + LαΔT
L' = 1.56392 + (1.56392) (19 x 10⁻⁶) (142)
L' = 1.56814 m
T' = New time period
New time period is given as
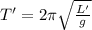

T' = 2.5134 sec
Change in time period is given as
ΔT = T' - T
ΔT = 2.5134 - 2.51
ΔT = 0.0034 sec