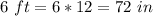Question

Bob fenced in his backyard. The perimeter of the yard is 22 feet, and the length of his yard is 5 feet. Use the perimeter formula to find the width of the rectangular yard in inches:

P = 2L + 2W.

Answer 1

The width of the rectangular yard is 72 inches

Explanation:

Let

L -----> the length of the yard

W ----> the width of the yard

we know that

The perimeter is equal to

`P=2(L+W)`

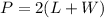
we have

`P=22\ ft`

`L=5\ ft`

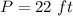
substitute in the formula and solve for W

`22=2(5+W)`

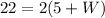
Divide by 2 both sides

`11=(5+W)`

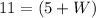
Subtract 5 both sides

`W=11-5=6\ ft`

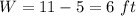
Convert feet to inches

Remember that

1 ft= 12 in

`6\ ft=6*12=72\ in`