Question

Please ASAP
20 points

Answer 1

1/2(y2-y1)(x3-x1). It must be choice D

Answer 2

`(1)/(2)(y_2-y_1)(x_3-x_1)`

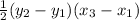
That looks like choice D.

Explanation:

I guess you are trying to find the area?

This is a triangle.

The formula for the area of a triangle is given as:

`(1)/(2)(\text{base})(\text{height})`.

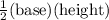So I see a line segment from a vertex of the triangle such that I can form perpendicular line segments so I can determine the base and height to use to find the area.

In my picture, I accidentally cut the word height off but the height is represented by that purple line segment.

The distance between the base and the vertex (or the length of the purple line segment) is
`x_3-x_1`. (Here I looked at the x's because it was a horizontal distance.)

The base length is in green. The distance between
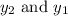`y_2 \text{ and } y_1` would give us the length which is
`y_2-y_1`. (Here I looked at the y's because it was a vertical distance.)

So anyways let's plug into our formula for the area of a triangle:

`(1)/(2)(\text{base})(\text{height})`

`(1)/(2)(y_2-y_1)(x_3-x_1)`

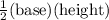
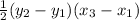
That looks like choice D.