Question

You need to prepare 100.0 mL of a pH 4.00 buffer solution using 0.100 M benzoic acid (p????a=4.20) and 0.240 M sodium benzoate. How many milliliters of each solution should be mixed to prepare this buffer?

Answer 1

Step-by-step explanation:

As it is given that
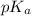`pK_(a)` = 4.20 and pH is 4.0.

Now, we use Henderson-Hasslbach equation as follows.

pH =
`pK_(a) + log ([salt])/(acid)`

So, it is given that salt is sodium benzoate and acid is benzoic acid. Hence, the given equation will become as follows.

pH =
`pK_(a) + log \frac{[\text{sodium benzoate}]}{\text{[benzoic acid]}}` ........ (1)

Now, substitute the given values into equation (1) as follows.

pH =
`pK_(a) + log \frac{[\text{sodium benzoate}]}{\text{[benzoic acid]}}`

4.0 =
`4.20 + log \frac{[{C_(6)H_(5)COONa}]}{[C_(6)H_(5)COOH]}`

`log \frac{[{C_(6)H_(5)COONa}]}{[C_(6)H_(5)COOH]}` = 4.0 - 4.20

= - 0.20

`\frac{[{C_(6)H_(5)COONa}]}{[C_(6)H_(5)COOH]}` =
`10^(-0.20)`

= 0.613

or,
`[C_(6)H_(5)COONa]` =
`0.613 * [C_(6)H_(5)COOH]` ............. (2)

Since, the total volume of (acid + base) is given as 100.0 mL. So, let us assume volume of acid is x and volume of base is y.

Hence, x + y = 100 mL ....... (3)

And, equation (2) will become as follows.

`y * 0.240 M` =
`0.613 * x * 0.1 M`

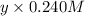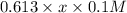
y = 0.255x

Substituting value of y into equation (3) as follows.

x + 0.255x = 100 mL

1.255 x = 100 mL

x = 79.68 mL

So, value of y will be as follows.

y = 0.255 x

= 0.255 × 79.68 mL

= 20.31 mL

Thus, we can conclude that volume of benzoic acid is 79.68 mL and volume of sodium benzoate is 20.31 mL.