Question

Two people start biking from the same point. One bikes east at 9 mph, the other south at 28 mph. What is the rate at which the distance between the two people is changing after 10 minutes and after 55 minutes

Answer 1

(a) 31.75 mph

(b) 31.77 mph

Step-by-step explanation:

(a) For t = 10 min = 10 / 60 = 1 / 6 hour

According to the question,

dx /dt = 15 mph

dy / dt = 28 mph

After 10 minutes

x = 1/6 x 15 = 2.5 miles

y = 1/6 x 28 = 4.67 miles

`d = \sqrt{x^(2)+y^(2)}=\sqrt{2.5^(2)+4.67^(2)} = 5.3 miles`

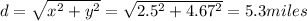
According to diagram

`D^(2) = {x^(2)+y^(2)}`

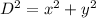
Differentiate both sides with respect to t.

2D dD/dt = 2 x dx/dt + 2y dy/dt

D dD/dt = x dx/dt + y dy/dt

5.3 dD/dt = 2.5 (15) + 4.67 (28)

dD/dt = 31.75 miles/hour

(b) For t = 55 minutes = 55 / 60 hours

After 55 / 60 hours

x = 55 / 60 (15) = 13.75 miles

y = 55 / 60 (28) = 25.67 miles

`d = \sqrt{x^(2)+y^(2)}=\sqrt{13.75^(2)+25.67^(2)} = 29.12 miles`

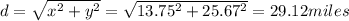
According to diagram

`D^(2) = {x^(2)+y^(2)}`

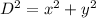
Differentiate both sides with respect to t.

2D dD/dt = 2 x dx/dt + 2y dy/dt

D dD/dt = x dx/dt + y dy/dt

29.12 dD/dt = 13.75 (15) + 25.67 (28)

dD/dt = 31.77 miles/hour