Question

Find the required annual interest rate to the nearest tenth of a percent for $1,400 to grow to $1,800 if interest is compounded quarterly for 9 yr.

Answer 1

Explanation:

For this problem, you should use the equation
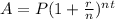`A=P(1+(r)/(n) )^n^t`. For this problem, A=1800, P=1400, n=4, r=x, t=9.

A = Final Amount

P = Principal (Original Amount)

n = Number of times it is compounded in a year. (Quarterly = 4)

r = Interest Rate (In the equation, you must convert your final answer to percent form)

t = Amount of years

Answer 2

the interest rate required is r = 2.8 % compounded quarterly.

Explanation:

principle (P) = $1,400

Grow to (A) = $1,800

compounded quarterly hence time = 9 year

= 9 × 4 = 36

rate will be equal to r/4

now,

`A =P(1+(r)/(100))^t`

`1800 =1400(1+(r)/(400))^(36)`

`ln (1.29) = 36 ln (1+(r)/(400))`

r = 2.8 %

hence, the interest rate required is r = 2.8 % compounded quarterly.