Answer:
3.974 Joule
Step-by-step explanation:
Diameter of ring = 7.7 cm
a = Distance from the center = d/2 = 3.85 cm = 0.0385 m
Q = Charge = 5 mC
q = Charge to move = 3.4 mC
k = Coulomb constant = 9×10⁹ Nm²/C²
Work done will be equal to Potential energy when mass is at center
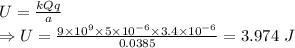
∴ Work to move a tiny 3.4 mC charge from very far away to the center of the ring is 3.974 Joule