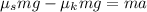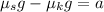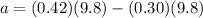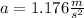Question

A student needs to move a large carton of books with a total mass of 120 kg, to get a better view out the window. The coefficient of static friction (μs) between the cardboard boxes and the tile floor is 0.42, and the coefficient of kinetic friction (μk) is 0.30. Assuming the student keeps pushing with the same force needed to get the box moving, what will be the acceleration of the box of books?

Answer 1

`1.176 (m)/(s^(2))`

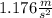
Step-by-step explanation:

`m` = Total mass of carton of books = 120 kg

`\mu _(s)` = Coefficient of static friction = 0.42

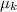`\mu _(k)` = Coefficient of kinetic friction = 0.30

`F` = force applied to get the box moving

`f` = kinetic frictional force acting on the carton of books

`a` = acceleration of the box

Force applied to get the box moving is given as

`F = \mu _(s)mg`

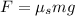
kinetic frictional force is given as

`f = \mu _(k)mg`

Force equation for the motion of the box of books is given as

`F - f = ma`

`\mu _(s)mg - \mu _(k)mg = ma`

`\mu _(s)g - \mu _(k)g = a`

`(0.42)(9.8) - (0.30)(9.8) = a`

`a = (0.42)(9.8) - (0.30)(9.8)`

`a = 1.176 (m)/(s^(2))`