Answer:

Step-by-step explanation:
Due to uniform electric field net flux through a closed surface is always zero
so here we have to find the net flux through a sphere due to a point charge placed at origin and due to a uniform electric field
so here we will have
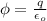
now we have
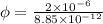

Due to external uniform field the electric flux is zero
so the total flux is only due to charge and it is equal to
