Question

Four cash prizes ($5000, $2000, $500 and $250) to be awarded to 4 students selected randomly from 15 students ( 7 from UCF and 8 from UF). In how many ways the prizes can awarded?

Answer 1

32760 ways

Explanation:

There are overall 15 students from which we have to select 4 students at random for the 4 Cash prizes.

i) First Prize can be distributed in
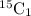`^(15)\textrm{C}_(1)` ways .

Also here we know the identity which says that

`^(m)\textrm{C}_(1)=m`

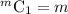
Hence
`^(15)\textrm{C}_(1)=15`

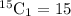
Now we are left with 14 students for second prize.

ii) Second prize can be distributed in
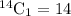`^(14)\textrm{C}_(1)=14` ways

Now we are left with 13 students for third prize.

iii) Third prize can be distributed in
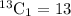`^(13)\textrm{C}_(1)=13` ways.

Now we are left with only one prize and which can be distributed among 12 Students

iv) Fourth prize can be distributed in
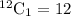`^(12)\textrm{C}_(1)=12` ways.

Hence total number of ways of distributing 4 prizes among 15 students is

`15 * 14* 13* 12`

= 32760