Question

A 0.30-kg flying pig toy is attached to the ceiling with a string. When the pig’s wings flap, it moves at a constant speed of � = 2.00 m/s in a horizontal circle of radius � = 0.25 m. Find the angle � the string makes with the vertical.

Answer 1

58.5 deg

Step-by-step explanation:

`v` = mass of the pig toy = 0.30 kg

`v` = speed of the toy = 2 m/s

`r` = radius of the circle = 0.25 m

`\theta` = Angle of the string with the vertical = ?

`T` = Tension force in the string

Using equilibrium of force in vertical direction

`T Cos\theta = mg` eq-1

Along the horizontal direction, the component of tension force provides the necessary centripetal force, hence

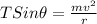`T Sin\theta = (mv^(2))/(r)` eq-2

Dividing eq-2 by eq-1

`(T Sin\theta)/(T Cos\theta) = ((mv^(2))/(r))/(mg)`

`tan\theta= (v^(2))/(rg)`

`tan\theta= ((2)^(2))/((0.25)(9.8))`

`tan\theta = 1.63`

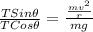
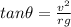
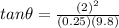
`\theta` = 58.5 deg