Question

An object is taken out of a 65 degree F room and placed outside where the temperature is 35 degree F. Five minutes later the temperature is 63 degree F. It cools according to Newton's Law. Determine the temperature of the object after one hour. Round to the nearest tenth of a degree.

Answer 1

the temperature of the object after one hour is 48.1 degree F

Step-by-step explanation:

Given data

room temp = 65 F

outside temp = 35 F

5 min temperature = 63 F

to find out

the temperature of the object after one hour

solution

according to Newton's Law

dT/dt = -K( T - 35)

dT/T-35 = -k dt

integrate both side

ln (T-35 ) = -kt +c

we take here T(0) = 65

ln (65 - 35) = -kt +c

so c = ln(30)

so it will be

ln (T-35 ) = -kt + ln30 .....................1

now we again take T(5) = 63 according to question

ln (63-35 ) = -kt + ln30

ln 28 = -5k + ln 30

so 5k = ln (30/28)

k = 1/5 ln(15/14)

now put this k value in equation 1

ln (T-35 ) = -1/5 ln(15/14)t + ln30

T-35 = 30
`(15/14)^(-t/5)`

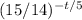
so after 1 hour temperature will be

T(60) = 30
`(15/14)^(-t/5)` +30

T(60) = 30
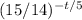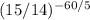`(15/14)^(-60/5)` +30

T(60) = 35 +30
`(15/14)^(-12)`

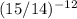
T(60) = 48.1 degree F

the temperature of the object after one hour is 48.1 degree F