Given:
Probability of winning, P(X) = 0.02
Probability of losing, P(
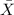 ) = 0.98
) = 0.98
Wining amount = $160
Losing amount = $16
Explanation:
Let the expected amount of money win be 'X'
Expected value of X, E(X) = Probability of winning, P(X).Probability of winning, P(X) - Probability of losing, P(
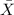 ).Losing amount
).Losing amount
Now,
E(X) = (
 )
)
E(X) = -12.48
Expected value of X = -12.48
Expected loss value = $12.48 loss