Question

An alpha particle (q = +2e, m = 4.00 u) travels in a circular path of radius 5.99 cm in a uniform magnetic field with B = 1.43 T. Calculate (a) its speed, (b) its period of revolution, (c) its kinetic energy, and (d) the potential difference through which it would have to be accelerated to achieve this energy.

Answer 1

a)
`4.1* 10^(6) (m)/(s)`

b)
`9.2* 10^(-8) s`

c)
`5.6* 10^(-14) J`

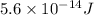
d) 175000 volts

Step-by-step explanation:

a)

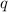`q` = magnitude of charge on the alpha particle = 2 x 1.6 x 10⁻¹⁹ C = 3.2 x 10⁻¹⁹ C

`m` = mass of alpha particle = 4 x 1.67 x 10⁻²⁷ kg = 6.68 x 10⁻²⁷ kg

`r` = radius of circular path = 5.99 cm = 0.0599 m

`B` = magnitude of magnetic field = 1.43 T

`v` = speed of the particle

Radius of circular path is given as

`r = (mv)/(qB)`

`0.0599 = ((6.68* 10^(-27))v)/((3.2* 10^(-19))(1.43))`

`v = 4.1* 10^(6) (m)/(s)`

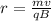
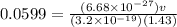
b)

Time period is given as

`T = (2\pi m)/(qB)`

`T = (2(3.14)(6.68* 10^(-27)))/((3.2* 10^(-19))(1.43))`

`T = 9.2* 10^(-8) s`

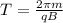
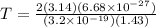
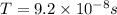
c)

Kinetic energy is given as

`K = (0.5)mv^(2)`

`K = (0.5)(6.68* 10^(-27))(4.1* 10^(6))^(2)`

`K = 5.6* 10^(-14) J`

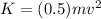
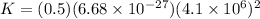
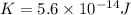
d)

ΔV = potential difference

Using conservation of energy

q ΔV = K

(3.2 x 10⁻¹⁹) ΔV = 5.6 x 10⁻¹⁴

ΔV = 175000 volts