Question

Suppose a baseball team has 14 players on the roster who are not members of the pitching staff. Of those 14 players, assume that three have recently taken a performance-enhancing drug. Suppose the league decides to randomly test five members of the team. What is the probability that at least one of the tested players is found to have taken a performance-enhancing drug

Answer 1

Answer: Our required probability would be 0.70.

Explanation:

Since we have given that

Number of players = 14

Number of players have recently taken a performance enhancing drug = 3

Number of players have not recently taken a performance enhancing drug = 14-3=11

Number of members chosen randomly = 5

We need to find the probability that at least one of the tested players is found to have taken a performance enhancing drug.

P(Atleast 1) = 1 - P(none is found to have taken a performance enhancing drug)

So, P(X≥1)=1-P(X=0)

`P(X\geq 1)=1-^5C_0((11)/(14))^5\\\\P(X\geq 1)=1-(0.786)^5\\\\P(X\geq 1)=0.70`

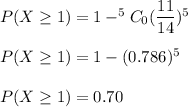
Hence, our required probability would be 0.70.