Question

g, Assume that random guesses are made for 15 multiple chioce questions on an SAT test and that there are 5 choices on each question with the probability of success 0.20. Find the probability that the number of correct answers is at most 6 (This problem meets all the requirements of a binomial situation.)

Answer 1

Answer: 0.9819

Explanation:

Binomial probability formula :-

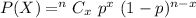`P(X)=^nC_x \ p^x\ (1-p)^(n-x)`, where P(x) is the probability of getting success in x trials, n is total number of trials and p is the probability of getting succes in each trial.

Given : The probability of success :
`p=0.20`

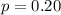
The total question answered : n= 15

Now, the probability that the number of correct answers is at most 6 is given by :-

`P(x\leq6)=P(0)+P(1)+P(2)+P(3)+P(4)+P(5)+P(6)\\\\= ^(15)C_(0)(0.20)^0(0.8)^(15)+^(15)C_(1)(0.20)^1(0.8)^(14)+^(15)C_(2) (0.20)^2(0.8)^(13)+^(15)C_(3) (0.20)^3 (0.8)^(12)+^(15)C_(4) (0.20)^4(0.8)^(11)+^(15)C_(5) \ (0.20)^5\ (0.8)^(10)+^(15)C_(6) \ (0.20)^6\ (0.8)^(9)\\\\=(0.8)^(15)+(15)(0.2)(0.8)^(14)+105(0.2)^(2)(0.8)^(13)+455(0.2)^(3)(0.8)^(12)+1365(0.2)^(4)(0.8)^(11)+3003(0.2)^(5)(0.8)^(10)+5005(0.2)^(6)(0.8)^(9)=0.981941193015\approx0.9819`

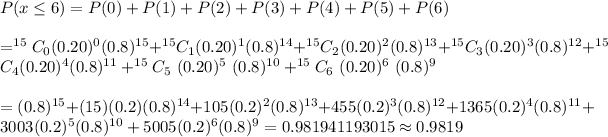
Hence, the probability that the number of correct answers is at most 6 = 0.9819