Answer:
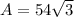
Explanation:
here we are going to use the formula which is
Area=
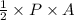
Where P is perimeter and A is apothem
Please refer to the image attached with this :
In a Hexagon , there are six equilateral triangle being formed by the three diagonals which meet at point O.
Consider one of them , 0PQ with side a
As Apothem is the Altitude from point of intersection of diagonals to one of the side. Hence it divides the side in two equal parts . hence
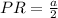
Also OP= a
Using Pythagoras theorem ,
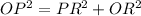
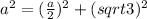
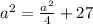
Subtracting both sides by
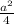
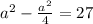
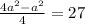
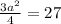
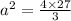

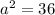
taking square roots on both sides we get
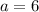
Now we have one side as 6 mm
Hence the perimeter is
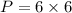
 mm
mm
Apothem =
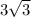
Now we put them in the main formula
Area =

Area=
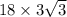
Area=
