Answer:
i = 3.18 A anticlockwise
Step-by-step explanation:
magnetic field due to wire at centre
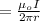
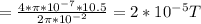
Current in the loop can be determined by using magnetic field formula in centre of loop
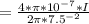
total net magnitude is given as 6.7*10^[-6} T
SO WE HAVE

solving above equation we get
i = 3.18 A anticlockwise to have magnetic field direction out of the page