Question

Based on a​ survey, assume that 28​% of consumers are comfortable having drones deliver their purchases. Suppose that we want to find the probability that when five consumers are randomly​ selected, exactly three of them are comfortable with delivery by drones. Identify the values of​ n, x,​ p, and q.

Answer 1

Answer with explanation:

We know that the formula for binomial probability :-

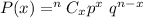`P(x)=^nC_xp^x\ q^(n-x)`, where P(x) is the probability of getting success in x trials , n is the total number of trials and p is the probability of getting success in each trial.

Given : The probability that consumers are comfortable having drones deliver their purchases = 0.28

The total number of consumers selected = 5

To find the probability that when five consumers are randomly​ selected, exactly three of them are comfortable with delivery by drones , we substitute

n=5, x=3 , p=0.28 and q=1-0.28=0.72 in the above formula.

`P(3)=^5C_3(0.28)^3\ (0.72)^(2)\\\\10(0.28)^3(0.72)^(2)\approx0.1138`

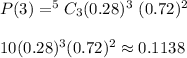
Thus, the probability that when five consumers are randomly​ selected, exactly three of them are comfortable with delivery by drones = 0.1138