Answer:
0.1 rev/s
Step-by-step explanation:
M = mass of the merry go round = 200 kg
R = radius of merry go round = 6 m
 = Moment of inertia of merry go round = (0.5) MR² = (0.5) (200) (6)² = 3600 kgm²
= Moment of inertia of merry go round = (0.5) MR² = (0.5) (200) (6)² = 3600 kgm²
m = mass of the man = 100 kg
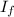 = Moment of inertia of merry go round when man sits on it at the edge = (0.5) MR² + mR² = (0.5) (200) (6)² + (100) (6)² = 7200 kgm²
= Moment of inertia of merry go round when man sits on it at the edge = (0.5) MR² + mR² = (0.5) (200) (6)² + (100) (6)² = 7200 kgm²
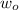 = initial Angular speed of merry-go-round before man sit = 0.2 rev/s
= initial Angular speed of merry-go-round before man sit = 0.2 rev/s
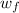 = Angular speed of merry-go-round after man sit = ?
= Angular speed of merry-go-round after man sit = ?
Using conservation of angular momentum

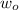 =
=
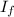
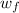
(3600) (0.2) = (7200)
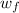
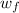 = 0.1 rev/s
= 0.1 rev/s