Answer:
E=
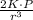
Step-by-step explanation:
We are given that a dipole consist of two charge Q and -Q and charge separated by l.Let a charge +1 C is placed at point P at distance r from the centre of dipole.
We have to find the magnitude of the electric field at point along the axis of dipole .
We know that Electric field=
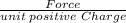
Electric filed due to positive charge Q
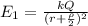 {from A to P}
{from A to P}
Electric field due to negative charge -Q
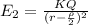 ( Along PB)
( Along PB)
Net electric field E=
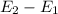
E=

E=
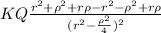
E=

We are given that r >> L then
E=
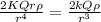
E=
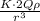
E=
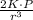
Where, P=Diple = Distance between two charges
 any charge
any charge