Question

A proton initially at rest is accelerated by a uniform electric field. The proton moves 4.76 cm in 1.10 x 10^-6 s. Find the voltage drop through which the proton moves.?

Answer 1

The voltage drop through which the proton moves is 39.1 V.

Step-by-step explanation:

Given that,

Distance = 4.76 cm

Time
`t=1.10*10^(-6)\ s`

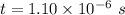
We need to calculate the acceleration

Using equation of motion

`s = ut+(1)/(2)at^2`

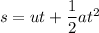
Where, s = distance

a = acceleration

t = time

Put the value in the equation

`4.76*10^(-2)=(1)/(2)* a *(1.10*10^(-6))^2`

`a=(2* 4.76*10^(-2))/((1.10*10^(-6))^2)`

`a=7.87*10^(10)\ m/s^2`

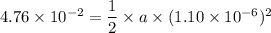
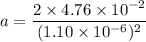
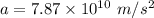
We need to calculate the voltage drop

Using formula of electric field

`F=qE`

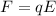
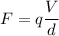`F = q(V)/(d)`....(I)

Using newton's second law

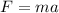`F = ma`....(II)

Put the value of F in equation (I) from equation (II)

`ma=(qV)/(d)`

`V=(mad)/(q)`

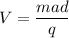
Where, q = charge

a = acceleration

d = distance

m= mass of proton

Put the value into the formula

`V=(1.67*10^(-27)*7.87*10^(10)*4.76*10^(-2))/(1.6*10^(-19))`

`V=39.1\ V`

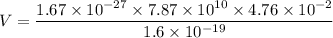
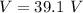
Hence, The voltage drop through which the proton moves is 39.1 V.