Answer:
Explanation:
Given that the Rockwell hardness of a metal is determined by impressing a hardened point into the surface of the metal and then measuring the depth of penetration of the point.
Let X be the hardness
X is N(68,3)
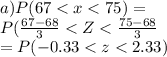
This area lies on both sides of the mean. Hence we add the corresponding prob values form table
= 0.1293+0.4901=0.6194